Last year a You-Tube video done by a west-coast weather forcaster made the rounds of the homeschooling virtual world.
 The weather forcaster started with the complaint that when she went back to school to study meteorology, she noticed that the math skills of her young colleagues was lacking and that this limited their ability to be successful in their studies. Thus far her argument holds up. It would be difficult to be successful in any scientific field if math skills are lacking.
The weather forcaster started with the complaint that when she went back to school to study meteorology, she noticed that the math skills of her young colleagues was lacking and that this limited their ability to be successful in their studies. Thus far her argument holds up. It would be difficult to be successful in any scientific field if math skills are lacking. But then the argument took an interesting turn. She said she went home and took a look at the methodologies that were being used to teach her children math. And she zeroed in (no pun intended) on three different methods used for multiplication. All three methods worked. That is a student could use any of the methods to calculate a correct answer. The argument came down to speed. She concluded that the problem her fellow students at the university level had with math was that they had been taught inefficient methodologies for problem solving, and so getting to the correct answer was taking more time. Another You-Tube video was made by a man who calculated exactly how much more time the alternative methodologies took and there was a difference of at least several seconds. The man did not calculate whether that difference was significant or not.
As I watched this You-Tube and the minor sensation it made in the homeschooling virtual world, I had a nagging sense that there was a real problem with the framing of the argument but I did not take the time to follow it through. Instead, I had flashbacks to third grade math with Mrs. C. With all the best intentions, Mrs. C. gave timed tests of multiplication and division. These were not timed tests of knowing the multiplication tables only. Instead, the people who did multi-step problems the fastest were considered math whizzes who would go on to be scientists and techno-geeks. Alas, I was not to be one of them. I was one of those kids who had poor motor skills and held my pencil too hard--which I still do when I am nervous--and so I never had the "whiz-kid" speed. And so I developed the idea that whereas I was good at reading, science, and history, I was no good at math. I continued to think so all the way through Algebra, Geometry and Algebra II and Trigonometry , Math Analysis and Pre-Calculus in high school.
I thought that I was no good in math, even though I got A's in my classes. I thought that I was no good in math even though I understood numbers and got very excited by ideas like negative numbers and scientific notation. I thought that I was no good in math right up to the point where I too the PSAT. Then I realized that there was something wrong with my assessment of my math ability. And when I found that I enjoyed calculus and what it could do for me in physics and chemistry and biology, I concluded that there was probably nothing wrong with my math abilities. (Yes--I am a Geek, first class. I also really enjoy statistics).
 I don't know how many people in Mrs. C.'s third grade math class became scientists. I do know that I did. And I now understand that when it comes to math, accuracy is far more important than speed. I don't know about you, but when I cross a highway bridge, my confidence in the engineering is based on a sense that the engineer can make accurate calculations. I really don't care if he did the problem seven seconds faster than someone else. Seven seconds is not really a very long time compared to the years that would be taken off my life if the bridge collapsed with me on it.
I don't know how many people in Mrs. C.'s third grade math class became scientists. I do know that I did. And I now understand that when it comes to math, accuracy is far more important than speed. I don't know about you, but when I cross a highway bridge, my confidence in the engineering is based on a sense that the engineer can make accurate calculations. I really don't care if he did the problem seven seconds faster than someone else. Seven seconds is not really a very long time compared to the years that would be taken off my life if the bridge collapsed with me on it. These are the thoughts that guide me as I go about re-teaching math to my son, N.
N. had a much more traumatic experience with math in third grade than I did. His fine-motor skills and hand-eye coordination were so compromised that he used to have great difficulty even lining up words or numbers corrrectly on a page. And mental math requires strong auditory working memory, another issue for N., who has Central Auditory Processing Disorder--a specific learning disability--on top of his AS diagnosis. You can imagine what happened when his teacher gave him timed math problems. We were told that N. did not know his math facts and that he was "slowing the class down." The third grade teacher added insult to injury by labeling N. publically as "lazy." It does not take a rocket scientist to conclude that N. was not motivated to actually learn math.
When I undertook to teach him math last year, I found that there were big holes in his understanding of basic operations. It was clear that he had missed important steps and concepts in his elementary school math training. This probably springs from two sources. One is that he had so much difficulty following verbal instructions that he simply missed much of what was being "taught." (My very wise professor in Special Education Assessment says that if a student hasn't learned something, the first hypothesis ought to be that he was not taught it. Covering material for a student is not the same thing as teaching that student). The second problem with math instruction in public schools is that, in their frantic urgency to improve standardized test scores, districts tend to change curricular programs very often. Program changes in the middle of someone's education tend to create gaps in learning because each program builds on previous instruction in different ways.
The other problem we face in order to re-teach math to N., is the issue of motivation. When N. sits down to do math, his anxiety climbs quickly to the red zone. He has learned that he is "no good at math." A large part of that problem has to do with speed. He has been inadvertently taught that if he cannot come up with the right answer in 15 seconds, then he is hopelessly stupid and there is no point in continuing.
Aside: In my opinion, this problem extends far beyond N. and far beyond math. I believe that one reason our schools are failing is that we have taught our kids that filling in the blank or the bubble for the "right" answer in all areas is far more important than actually thinking something through.
But enough! Back to the main story.
We have developed the following principles for re-teaching N. math.
1. Teach basic math from the beginning.
When N. expressed the desire to learn algebra, I took him out for coffee and we had a conversation about math instruction. I explained to him what I had observed about how poorly math is taught in elementary school in general, and what was lacking in his math instruction in particular. I listened while he told me about the feelings of fear and anxiety he has whenever he sits down to a math problem. Then I told him that it was my hypothesis that if he started math from the beginning operations and systematically studied them, we could certainly be doing algebra by next year.
We made an agreement about how we would help N. reach his goal. So that N. could learn from the beginning of the operations, we ordered Basic Math from the Teaching Company's Great Courses. We also agreed that we would not be concerned about time. N. would proceed through the material systematically, taking all of the time he needs to learn each operation and concept well. And we agreed that would work on auditory working memory outside of math i.e. there would be no verbal drills. And this all leads to:
2) Speed is not important, accuracy is.
Our goal at present is that N. should use a variety of methods in order to learn algorithms and to understand the number theory underlying them. Just as we are not worried about how long it takes for N. to learn the math, so we are also not concerned about how long it takes to do a problem set.
 We have taught N. a new mantra: "This is why pencils have erasers." Since accuracy is more important than speed to us at this point, it would be easy to fall into the trap of thinking that errors are fatal. But at this point, we are far from designing bridges and airplanes, the important point here is that N. learn to check his answers and learn to find and correct his mistakes. To do that, N. must understand the concepts that underlie the algorithms he is learning. When he comes to apply math to things like bridge design, it will be very important that he can check his answers for himself and be able to know that they are accurate.
We have taught N. a new mantra: "This is why pencils have erasers." Since accuracy is more important than speed to us at this point, it would be easy to fall into the trap of thinking that errors are fatal. But at this point, we are far from designing bridges and airplanes, the important point here is that N. learn to check his answers and learn to find and correct his mistakes. To do that, N. must understand the concepts that underlie the algorithms he is learning. When he comes to apply math to things like bridge design, it will be very important that he can check his answers for himself and be able to know that they are accurate. I know, I know! What about standardized testing? That is the big question that the Math Speed Demons out there will definitely be asking. Isn't speed important to those?
Answer: Yes, in a way. Actually, what those tests require is familiarity with the basic operations to the point of automaticity. That means that the student is able to add, subtract, multiply, and divide without needing to develop the algorithm each time. This allows the real problem-solving power to be applied to more complex math using algebra and calculus. And here is the take-home message to this idea:
When a student is learning to understand math operations and develop algorithms, he will be slower at working problems. As the algorithms become automatic, the speed will increase.
Think about it. When you take it upon yourself to learn a complex skill, it goes slowly at first. As your brain builds the needed neural connections, your speed and accuracy improve. Eventually, you have made certain skills automatic and now they can be applied to more complex problems. For example, when I first started to embroider, I did not know any of the stitches. So my first project took what seemed like forever, as I learned the basic stitches needed on each part of the project. The more projects I did, the faster I completed them, until I was able to do large and ambitiously complex projects with enough speed to actually enjoy them.
 By going back to fill in the gaps for both knowledge and skills without concern about speed, we hope that N. will "automate" the basic math operations. As his skill and accuracy improves, so will his timing.
By going back to fill in the gaps for both knowledge and skills without concern about speed, we hope that N. will "automate" the basic math operations. As his skill and accuracy improves, so will his timing.But the ultimate goal is not to be fast! It is to appreciate numbers and enjoy applying math to real-world problems in order to get good results and satisfying answers to the big questions.


















12 comments:
Great post--I'll link.
Another brilliant post. Thank you. Timing was excellent as I was complaining about math again to my husband this weekend.
Awesome post, Elisheva!
When S. left public school in 4th grade, I was surprised at the huge gaps in her math learning. She also has (C)APD & AS, and she also has NVLD (Non Verbal Learning Disability) which complicates visual spatial and mathematical learning. Her eager teachers had pulled her through third grade and managed to get her a "Proficiency" rating on her 3rd grade Math SOLs (the standardized tests which - along with Bush's brainchild, No Child Left on the Playground - are the focus of education in our state) She was doing multi-digit addition and subtraction with regrouping, but didn't grasp place value at all. She'd gained some poorly learned rote skills with no comprehension. With the help of Marilyn Burns, I realized many neurotypical kids run into this problem, too. We did a lot of "backtracking," and are still struggling now.
I'd like to post about this soon, when I can get focused. I'd like to link to this post, too.
This is a fine post. Math has always been the hardest subject around here. I carry my own math anxiety and have covered only a fraction of the math you seem to know. My oldest is now doing OK in high school algebra but in his rush to the answer he often skips steps and does sloppy work. My younger son *hates* math and his distaste for the subject makes it that much harder to keep at it regularly and make progress. My 5 yo daughter still loves numbers and all I can do is hope to keep that love alive.
Very interesting!
We went back a year when T. started 3rd grade to Singapore 2nd grade books because I felt like he needed a better foundation. He is now fairly confident in his abilities (though he still adds on his fingers And Toes, which makes me crazy).
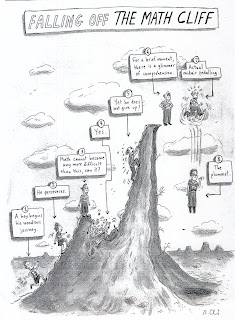
I love the cartoon! That was me and math -- I fell off the cliff when it came to college statistics.
What a great post!!
We are taking a break with my 3rd grader in math to focus on addition and subtraction facts. I ordered several math games from Rainbow Resource and Mary is asking every day, "Is my stuff here yet?"
Even with homeschooling only a few children I have managed to find gaps in their math skills- that after using the same program since K. I can't imagine what they would have been like being in a chaotic classroom with 30 other kids.
Great post and great point -- accuracy is more important than speed. Maybe in my case the point would be accuracy is at least as important as concepts. I was ahead of the math game in jr. high, taking algebra in 8th grade, but failed it -- I had never even made a C before!-- not because i didn't understand it, but because I had not memorized the facts (x, +)and because I would mentally leap ahead and skip steps, thinking I could get away with that because I knew where the problem was going. When I repeated algebra, I was blessed to have a strict and meticulous teacher who required that we write out every single step, and would mark a problem wrong if we did not, even if the outcome was correct. This drove some of the students mad, but it was exactly what I needed.
Great post. I had a similar experience in school. I loved math but everyone kept telling me it wasn't my 'thing' because I was just too slow. I'm glad I have the opportunity to give my girls as much time as they need to become comfortable and competent in the world of numbers.
That is a wonderful post! I had a similar situation with a math teacher -who added insult to injury by telling us we better practice our waitressing skills when we didn't complete problems as fast as he would have liked. No need here, I ended up majoring in accounting!
Thank you! I sooo needed this post. I've been drilling my daughter thinking speed equaled good at math and yet when left to her own devices my daughter would plod through a sheet...But get everything right.
I need to chill out.
Hi,
Your post was very touching.
I've always been very good at Math but certainly not the quickest at doing arithmetic.
A couple of comments that may be helpful to you and others:
1. Mathematical reasoning and doing arithmetic quickly are not the same skill. Arithmetic is largely an algorithmic mechanical process. Some people can mechanically process data more quickly than others. Others can do abstract reasoning better but not do so well at arithmetic. Einstein was a prime example of this. He could come up with brilliant formulas but then needed help to do the calculations.
2. Making arithmetic errors can have different causes and different solutions. I realize that making errors in calculation is different than being able to do arithmetic quickly.
I have a couple of articles I've written on my blog, WildAboutMath.com that may be of interest:
Good storytelling ability related to good mathematical skills. This article touches on how Math is different from arithmetic although it's not the main focus of the article.
How to get past "stupid" Math mistakes. This one discusses some possibilities for where errors in calculation come from and ways to address them.
Sol
I love the way you are working with you child since his fears and anxieties regarding math create a feeling isolation for him, but you fully demonstrated that he has a very important ally in you.
A great product to teach the automaticity of math facts is found at this site
www.AMatterofFacts.com
It will help your child learn their facts using arcade games while tracking specific trouble facts and reporting them to you in the form of activity sheets and flash cards. Your child will love it!
Post a Comment